The drivetrain of a motor vehicle is the group of components that deliver power to the driving wheels. This excludes the engine or motor that generates the power. In contrast, the powertrain is considered as including both the engine or motor, and the drivetrain.

For a motorcycle the rear wheel is also part of the power train as it delivers the power to the road.
So the size of the rear tire also affects the entire drive train ratio.

Gear Ratio

According to Wikipedia Gear Ratio is:
"The relationship between the number of teeth on two gears that are meshed or two sprockets connected with a common roller chain, or the circumferences of two pulleys connected with a drive belt."

But in a motorcycle the are more than one gear at work so there also are more gear ratio. All these are working together and form the overall ratio. Most gearing consists off:

• Primary drive, this is the gear ratio between engine RPM and the clutch shaft RPM, also called 'Primary reduction ratio'
• Gearbox ratio, this is the ratio between the clutch shaft RPM and the countershaft RPM. Because there are more gears in a gearbox, different ratio are possible
• Final drive ratio, this is the ratio between countershaft RPM and rear sprocket RPM
• The ratio between the Rear sprocket and the rear wheel (rear tire that is !)

Overall Gearing Ratio

The overall gearing of a motorcycle consists of 3 major parts:

1. The primary drive, the ratio between the engine (crankshaft) and the clutch or gearbox.
2. The gear box it self, depending on the amount of gears this part has an equal amount of ratio to choose from
3. The Final drive, (or secondary) ratio between the number of teeth on the Rear sprocket and the Front sprocket

All these ratio combined results in the Overall Gearing Ratio of the power train:

Changing any of these parts or changing the rear wheel tire's dimensions will affect speed and / or torque results. All of these changes can be simulated by using the GC by changing involved gearing fields. To be able to do this correct, all ratio, sizes and RPM's are mandatory input.

Primary Drive

The primary drive is the ratio between the engine's crankshaft and the incoming shaft of the gear box or clutch. Usually this ratio can not be easily altered unless you perform major surgery on your bike.... But if you do, don't forget to change the Primary Drive Ratio field in the GC accordingly.
 

The gear box

The gear box is the only gearing part that can change its ratio very easily and that is by you changing gear while you drive. Normally a gear box is dedicated for an engine or bike so you do not have to modify gear ratio in the gearbox itself. But sometimes the stock gears are not suited for all circumstances and manufacturers offer a way to change the internal gear box ratio by either offering different cogwheels or by offering a so called cassette gearbox that can be swapped easily. That is why in the GC you can change the gear ratio of each gear by changing the values in the red and blue gear box ratio fields for each gear.
 

The Final Drive and consequences of changing it

The final drive ratio is the last bit of gearing between your transmission and the driven wheel.  In our example the Final Drive ratio is the ratio of the final part of the gearing, the ratio between Rear and Front sprocket. This is also referred to as 'Secondary Ratio'.

Below a table containing the possible final drive gearing (ratio) changes and their results on Speed and Torque.

As a rule of thumb, changing the front sprocket with 1 tooth, amounts to the same effect as changing the rear sprocket with 3 teeth. This does not exactly apply to every bike but as a rule of thumb it will do and explains the results in the table below. 

As you can see, the effect of adding a front tooth and keeping the rear the same has about 3 times more influence on the speed than  removing 1 tooth in the rear and keeping front the same. Changing only the rear with 1 tooth does not have a lot of effect, you can use it to 'fine tune' the final drive. In combination with also changing the the front it either amplifies or weakens the total effect.
When you change more than just 1 tooth in front and/or rear, the results will increase significantly of course.

The exact increase or decrease in both % and actual speed for your bike can be calculated using the Gearing Commander tables.

Combining all these individual ratios results in the Overall power train ratio or the Overall ratio.
Just multiply all the ratio to get the Overall Ratio =  Primary ratio * Gear Box ratio *  Final Drive ratio

Rear wheel Tire Size changes and their consequences

As the rear tire is a major part of the total bike gearing, changing its size does impact this gearing and hence impact speed and torque at certain RPM's. This is easy to explain: the chain, belt or drive shaft is rotating the rear wheel at a certain RPM. Now when it is a small wheel in diameter it will have a small circumference and when rotated it will cover only a small distance. A bigger wheel will cover a bigger distance when rotated equally fast.

So changing for instance your rim size from 16" to 17" would mean a higher (top-)speed at the same RPM.
But not only changing the rim size influences gearing, also just changing the width of a tire changes gearing.
How come ? Well the tire circumference will change as there is a relation between the height of the tire and it's width:
The height of the tire is a percentage of the width. Also the height of the tire affects the circumference and hence the speed.

A modern tire has size marks on it like this '190 / 50 / 17' which means: the tire width is 190 mm, the rim diameter size is 17" and the height of the tire is 50% of the width ( 50% of 190 = 95 mm).

Let's asume you have an older bike (1982 Suzuki GS750T) which was originally fitted with a 4.5H17 4PR rear tire which is not available anymore and you want to replace it with a similar modern tire. The original tire has a width of 4.5" which is 114.3 mm. It's circumference was 2074.7 and theroretical top speed 208.1 Km/h. A similar wide modern tire could either be a 120/90/17 or a 130/80/17.

Though all 3 mentioned tires have the same rim size, as their width and heights are different, so will be their circumference:

4.5H17 4PR - width = 114.3 mm, circumference = 2074.7 mm, theoretical top speed: 208.1 Km/h
120 / 80 / 17 - width = 120 mm, circumference = 2035.1 mm, theoretical top speed: 204.1 Km/h
130 / 90 / 17 - width = 130 mm, circumference = 2010.0 mm, theoretical top speed: 201.6 Km/h

'Total' Gearing Ratio

As shown above, the tire size does affect the speed of the bike but it is not part of the commonly used 'Overall Gearing Ratio'. Say we want to compare 2 identical bikes, so having the same primary drive ratio, the same gearbox ratio and also the same final drive ratio but having rear tires with different sizes. This will resullt in an Overall Gearing Ratio which is the same for both bikes but they will perfom differently due to the different rear tire circumferences. So at a fixed RPM they will both reach different speeds.

The same 'difference in speed' could be achived by both bikes having the same rear tire size but giving them a different overall gearing ratio'. Now in order to see how much the rear tire affects the speed as opposed to the same bike with the stock rear tire, a new overall gearing ratio is calculated which incoporates the rear tire size ratio of both bikes.

Example Harley Davidson Softail FXSB Breakout '13-'16:
Stock  Bike  A:  Tire = 240/40/18, circumference = 80.30", speed @5600RPM = 152.6 Mph, Overall ratio = 2.791
Current Bike B: Tire = 260/40/18, circumference = 82.28", speed @5600RPM = 156.4 Mph, Overall ratio = 2.791

For the first bike (A) with the stock tire to get to 156.4Mph @5600 RPM, the overall gearing ratio would have to be

(RPM * Circumference) /  (Speed/min) =  (5600 * 80.3) / ((156.4 /60) * 63360) =  2.723

So the effect of having a different rear tire on bike 'B' as compared to the otherwise same bike 'A' is the same as changing the overall gearing ratio of bike 'A' from 2.791 to 2.723.

Incorporating the rear tire ratios into the overall ratio creates the 'Total Gearing Ratio'. This Total Ratio is also shown in the table "Overall and Total Ratio" which is calculated and shown when clicking the 'Overall & Total Ratio' button on the home page.

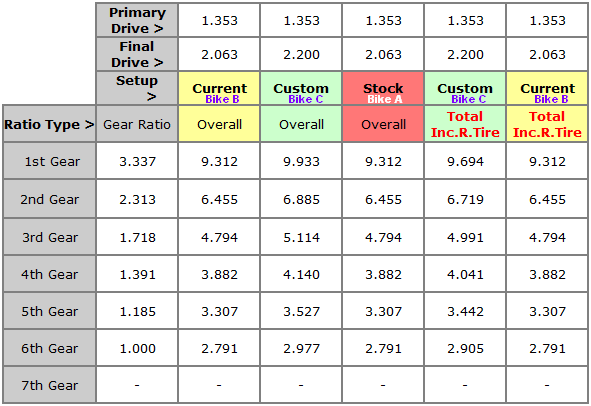
Now say Custom bike 'C' is like bike 'B' (so with the wider rear tire) and also has a smaller front sprocket, 30 instead of 32. This means the Overall Ratio will be different as the final drive changed and the Total Ratio will change because of the changed Overall Ratio and a changed rear tire.

Custom Bike C, Tire = 260/40/18, circumference = 82.28", speed @5600RPM = 146.6 Mph, Overall ratio = 2.977
By changing to a wider rear tire, the Total Ratio changed to 2.905

Top of page      More info on Tire Circumference

Back to Gearing Commander Main page